 Global Information
Global InformationAxiom of choice information
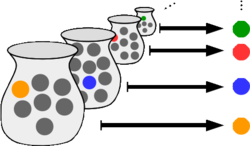

In mathematics, the axiom of choice, abbreviated AC or AoC, is an axiom of set theory equivalent to the statement that a Cartesian product of a collection of non-empty sets is non-empty. Informally put, the axiom of choice says that given any collection of sets, each containing at least one element, it is possible to construct a new set by choosing one element from each set, even if the collection is infinite. Formally, it states that for every indexed family of nonempty sets, there exists an indexed set such that for every . The axiom of choice was formulated in 1904 by Ernst Zermelo in order to formalize his proof of the well-ordering theorem.[1]
In many cases, a set created by choosing elements can be made without invoking the axiom of choice, particularly if the number of sets from which to choose the elements is finite, or if a canonical rule on how to choose the elements is available — some distinguishing property that happens to hold for exactly one element in each set. An illustrative example is sets picked from the natural numbers. From such sets, one may always select the smallest number, e.g. given the sets {{4, 5, 6}, {10, 12}, {1, 400, 617, 8000}}, the set containing each smallest element is {4, 10, 1}. In this case, "select the smallest number" is a choice function. Even if infinitely many sets are collected from the natural numbers, it will always be possible to choose the smallest element from each set to produce a set. That is, the choice function provides the set of chosen elements. But no definite choice function is known for the collection of all non-empty subsets of the real numbers. In that case, the axiom of choice must be invoked.
Bertrand Russell coined an analogy: for any (even infinite) collection of pairs of shoes, one can pick out the left shoe from each pair to obtain an appropriate collection (i.e. set) of shoes; this makes it possible to define a choice function directly. For an infinite collection of pairs of socks (assumed to have no distinguishing features), there is no obvious way to make a function that forms a set out of selecting one sock from each pair without invoking the axiom of choice.[2]
Although originally controversial, the axiom of choice is now used without reservation by most mathematicians,[3] and is included in the standard form of axiomatic set theory, Zermelo–Fraenkel set theory with the axiom of choice (ZFC). One motivation for this is that a number of generally accepted mathematical results, such as Tychonoff's theorem, require the axiom of choice for their proofs. Contemporary set theorists also study axioms that are not compatible with the axiom of choice, such as the axiom of determinacy. The axiom of choice is avoided in some varieties of constructive mathematics, although there are varieties of constructive mathematics in which the axiom of choice is embraced.
- ^ Zermelo 1904.
- ^ Jech 1977, p. 351
- ^ Jech, 1977, p. 348ff; Martin-Löf 2008, p. 210. According to Mendelson 1964, p. 201:
- The status of the Axiom of Choice has become less controversial in recent years. To most mathematicians it seems quite plausible and it has so many important applications in practically all branches of mathematics that not to accept it would seem to be a wilful hobbling of the practicing mathematician.



